Evaluation of the required Heating Power
The basic precondition for all inductive heating procedures is, that a metallic work piece with specified dimensions should be heated up in the time t to a predetermined temperature T.
The required heat quantity Q for heating up an object is calculated as follows:
Q = m x c x (T2 - T1)
Q heat quantity in kcal
m weight of the work piece in kg
c specific heat quantity in kcal/kg per degree
T1 initial temperature in °C
T2 final temperature in °C
Here are some conversion factors for various units:
Q x 4.18 [kWs]
Q x 0.07 [kWmin]
Q x 1.16x10-3 [kWh]
The specific heat c is a matter constant, and its value is added to a number of work materials.
If the heat quantity applied is determined according to the equation as shown above, care must be taken that the specific heat quantity c is not inserted as a constant in case of large differences of temperature. Furthermore, the latent heat of transformation to be furnished must also be taken into account from case to case. Power losses due to heat radiation and heat conduction are also neglected.
If the heating time is also considered, then the power in the work piece will be:
Pw=Q/t
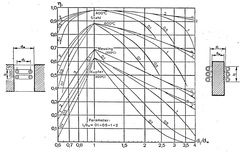
The efficiency of the inductor has a great influence on the required generator power. It may be considerably above the power required in the work piece. Its value may be approximately determined from the table shown below.
The required heat quantity Q for heating up an object is calculated as follows:
Q = m x c x (T2 - T1)
Q heat quantity in kcal
m weight of the work piece in kg
c specific heat quantity in kcal/kg per degree
T1 initial temperature in °C
T2 final temperature in °C
Here are some conversion factors for various units:
Q x 4.18 [kWs]
Q x 0.07 [kWmin]
Q x 1.16x10-3 [kWh]
The specific heat c is a matter constant, and its value is added to a number of work materials.
If the heat quantity applied is determined according to the equation as shown above, care must be taken that the specific heat quantity c is not inserted as a constant in case of large differences of temperature. Furthermore, the latent heat of transformation to be furnished must also be taken into account from case to case. Power losses due to heat radiation and heat conduction are also neglected.
If the heating time is also considered, then the power in the work piece will be:
Pw=Q/t
The efficiency of the inductor has a great influence on the required generator power. It may be considerably above the power required in the work piece. Its value may be approximately determined from the table shown below.
The required minimum working frequency is also important. It depends on the current penetration depth and on the material used.
It will also be calculated below.
Reference to Calculations in Case of Continuous Processing
If a work piece should be heated up in a continuous procedure, such as for instance a cable, then we must know its feed rate.
For instance:
Feed rate of cable: 15 m/min.
Let us assume the following values for the calculation:
Length = 15 m
Heating-up time = 1 minute
It will also be calculated below.
Reference to Calculations in Case of Continuous Processing
If a work piece should be heated up in a continuous procedure, such as for instance a cable, then we must know its feed rate.
For instance:
Feed rate of cable: 15 m/min.
Let us assume the following values for the calculation:
Length = 15 m
Heating-up time = 1 minute